Difference between revisions of "Biharmonic"
From MohidWiki
| Line 1: | Line 1: | ||
| − | The biharmonic filter is an extra term that is added | + | The biharmonic filter is an extra term that is added in the horizontal turbulent viscous flux, '''J''', that filters high frequency oscillations in a numerical model of advection-diffusion of a property <math>C</math>(i.e. oscillations with a wave-length equal to one or two widths of the horizontal resolution): |
<math>\mathrm{J} = -\mathrm{K}\cdot \nabla C + \nabla_{h}\left( \kappa_4 \nabla_{h}^2 C \right) </math> | <math>\mathrm{J} = -\mathrm{K}\cdot \nabla C + \nabla_{h}\left( \kappa_4 \nabla_{h}^2 C \right) </math> | ||
| + | where <math>\mathrm{K}</math> is Fick's law diffusivity. | ||
A ready made formula that would estimate the order of magnitude of the <math>\kappa_4</math> coefficient would be | A ready made formula that would estimate the order of magnitude of the <math>\kappa_4</math> coefficient would be | ||
Revision as of 14:28, 28 June 2011
The biharmonic filter is an extra term that is added in the horizontal turbulent viscous flux, J, that filters high frequency oscillations in a numerical model of advection-diffusion of a property 
(i.e. oscillations with a wave-length equal to one or two widths of the horizontal resolution):
where 
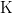is Fick's law diffusivity.
A ready made formula that would estimate the order of magnitude of the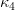 
coefficient would be
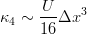
where U is a typical flow velocity and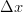 
is the numerical model horizontal resolution.
MOHID
In mohid, you need to add in the Hydrodynamic_x.dat the following keywords:
BIHARMONIC : [0/1] <-- Activates de biharmonic viscous flux BIHARMONIC_COEF : [Real] <-- Sets the value of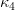
References
Delhez, E., & Deleersnijder, E. (2007). Overshootings and spurious oscillations caused by biharmonic mixing. Ocean Modelling, 17(3), 183-198. doi: 10.1016/j.ocemod.2007.01.002.
