Module PorousMediaProperties

Main Processes

Water flow

The water movement in soil will be dependent on the pressure gradients existing in the soil profile and also according to gravity. The equation that describes this motion is the Buckingham Darcy equation (Jury et al,1991) where J is the water velocity, h is the pressure head, θ is the water content and K is the hydraulic conductivity.

$J=-K\left ( \theta \right )\left ( \frac{\partial h}{\partial \theta } + 1 \right )$

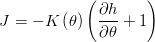
Water retention

The shape of water retention curves can be characterized by several models, one of them known as the van Genuchten model:

$\theta(h) = \theta_r + \frac{\theta_s - \theta_r}{\left[ 1+(\alpha |\psi|)^n \right]^{1-1/n}}$

where

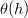$\theta(h)$ is the water retention curve [L³L⁻³];

Failed to parse (unknown error): |\h| is suction pressure ([L⁻¹] or cm of water);

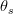$\theta_s$ saturated water content [L³L⁻³];

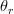$\theta_r$ residual water content [L³L⁻³];

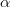$\alpha$ is related to the inverse of the air entry suction, $\alpha >0$ ([L⁻¹], or cm⁻¹); and,

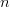$n$ is a measure of the pore-size distribution, 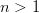$n>1$ (dimensionless).

Evapotranspiration

Some water may disappear from the soil because of the evaporation and transpiration processes, which become a sink in soil water profile. These two processes, currently named Evapotranspiration may be modeled using the Penmann Monteith equation.

$\overset{\text{Energy flux rate}}{\lambda_v E=\frac{\Delta R_n + \rho_a c_p \left( \delta q \right) g_a }{\Delta + \gamma \left ( 1 + g_a / g_s \right)}}~ \iff ~ \overset{\text{Volume flux rate}}{ET_o=\frac{\Delta R_n + \rho_a c_p \left( \delta q \right) g_a } { \left( \Delta + \gamma \left ( 1 + g_a / g_s \right) \right) \lambda_v }}$

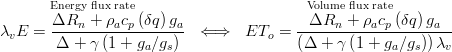
λ_v = Latent heat of vaporization. Energy required per unit mass of water vaporized. (J/g)

L_v = Volumetric latent heat of vaporization. Energy required per water volume vaporized. (L_v = 2453 MJ m^-3)

E = Mass water evapotranspiration rate (g s^-1 m^-2)

ET_o = Water volume evapotranspired (m³ s^-1 m^-2)

Δ = Rate of change of saturation specific humidity with air temperature. (Pa K^-1)

R_n = Net irradiance (W m^-2), the external source of energy flux

c_p = Specific heat capacity of air (J kg^-1 K^-1)

ρ_a = dry air density (kg m^-3)

δe = vapor pressure deficit, or specific humidity (Pa)

g_a = Hydraulic conductivity of air, atmospheric conductance (m s^-1)

g_s = Conductivity of stoma, surface conductance (m s^-1)

γ = Psychrometric constant (γ ≈ 66 Pa K^-1)

In Figure below it can be seen that the actual transpiration and evaporation are calculated in Porous Media module of MohidLand. The actual evaporation, which happens only at the soil surface, is calculated based on a pressure head limit imposed by the user. It allows the model to not evaporate any surface water, even if it is available, when the soil is very far way from the saturation point.

Evapotranspiration fluxogram in Mohid Land model